|
|
The BST is considered as a rigid cylinder.
Last updated by Thomas Naumann
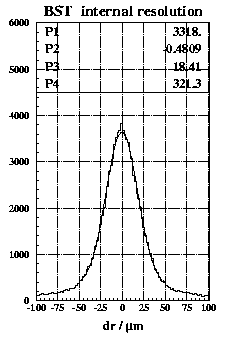
- an external alignment of the BST
in the H1 coordinate system
- an internal alignment of the disks
in the BST coordinate system .

The external position of the BST is characterized by:
- 3 translation parameters DR, DPHI, DZ and
- 2 rotation parameters THX and THY around the x and y
axes.
It is determined from the mispointing dz of a BST track to the
vertex
and checked with the difference dth of the polar angles measured
by BST and BDC.
Before alignment dz
and dth strongly depend on the azimuthal angle phi.
Assuming the BST gravicenter as the rotation center ZROT this
is parametrized as
DZ = (DR*COS(PHI-DPHI) + (THX*SIN(PHI)+THY*COS(PHI))*(Z-ZROT))* COT(TH) + DZ
The 5 external alignment constants are fitted from the
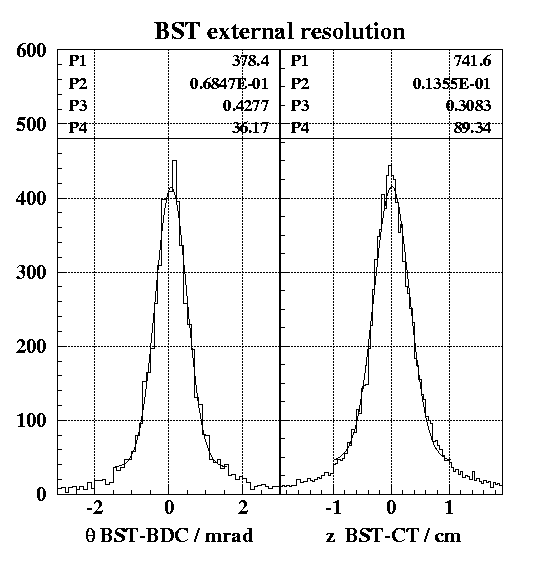
3D distribution dz(phi,z). We find
DR = 1.2 mm DZ = 0.5 mm THX = 1.2 mrad THY = 0.0 mrad
After external alignment no z or theta biases and no phi dependence
of dz
and dth
are visible. Integrating over phi we get
the z and theta resolutions of the
BST:
z BST - z CT = 0.01
+/- 0.31 mm
th BST - th BST = 0.07 +/- 0.43
mrad
The z
and theta
resolutions are also available for BST1 and BST2 separately.
dth is dominated by the BDC resolution.
dz contains 2 mm z error of the CT.
The external and internal alignment of
the r and u detectors is given
in the banks BSTA
and BSTU
.